Wire antennas are probably the first choice for portable use on the shortwave amateur radio bands.
I will show you on this webpage how to calculate a universal antenna tailored for several bands.
The starting point is a doublet antenna, because it consists of 2 radiator halves and a two-wire cable.
Since these antennas are not resonant, they require an antenna tuner and a balun to adapt the asymmetrical transceiver output to the symmetric feedline.
I wanted to build an antenna that could be used as universal as possible.
It should be used both with a feedline, for example, as an inverted-v and without a feedline as a vertical.
And I just wanted to build them for a few amateur radio bands and only make them as long as necessary.
If the length of the antenna wire and the feedline on a band is an integer multiple of ½ λ, a very high impedance occurs at the base.
And this high impedance usually can not adjust the antenna tuner.
However, since operation is desired on several bands, the search for lengths for the antenna wire and for the feedline begins, where only moderate foot point impedances occur on all bands.
Suitable connection points
Karl H. Hille, DL1VU, came up with the idea of calculate the appropriate location for the connection point of the feedline in the book "Windom- und Stromsummen-Antennen" [1].
In it, he calculates the optimal point on the resonant antenna wire of a Windom antenna.
But the method he used can also be applied to one half of a doublet antenna.
Because there is also a high-impedance at one end and a point with moderate impedance is sought.
However, the algorithm for calculating Windom and current sum antennas has a peculiarity that leads to errors if not observed.
When determining suitable contact points, the currents flowing at a certain point of the antenna of all bands are added.
This creates the characteristic "mountain range" as in the picture below.
In principle, only the summits (maxima) may be considered - side summits are not usable.
The making of the product is more meaningful.
However, for the product, the result is low even if the value on a radio band is low.
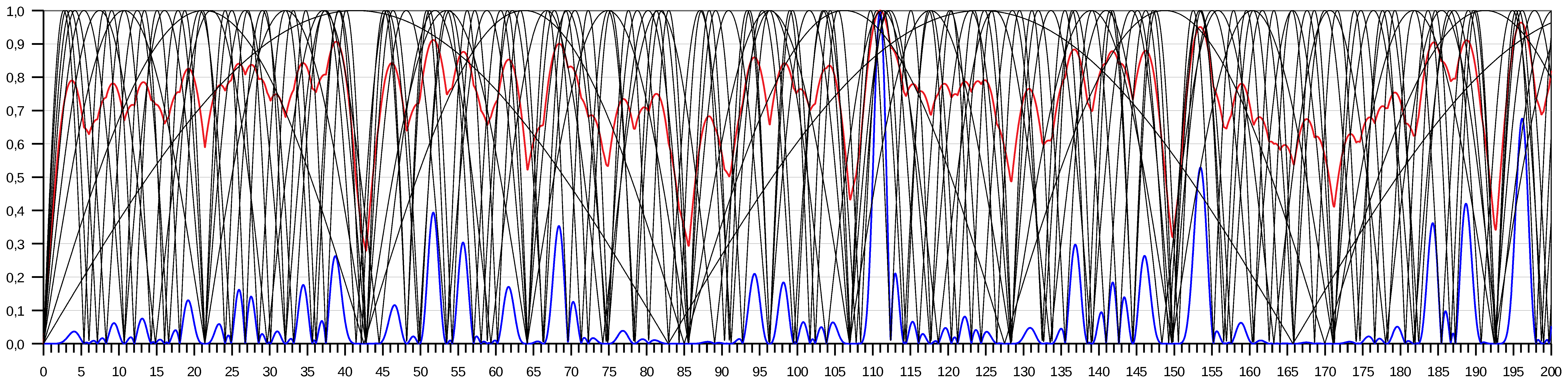
The picture above shows the current sum (red) and the current product (blue) on a 9-band antenna for 160 to 10 m to 2 00 m in length.
The maxima of the sum and the product are roughly in identical places.
But the usable maxima are more recognizable in the product.
The following form outputs the maxima up to a distance of 200 m from the high-impemature end of the wire with with values greater than 0.1.
Up to 9 bands and frequencies can be entered.
I was looking for an antenna that could be used for the CW transceiver Elecraft K1.
These transceiver is designed only for the 40-, 30-, 20- and 17-m-band, the antenna must also be sized only for these 4 bands.
The first 4 maxima are 5.51 m, 9.43 m, 12.58 m und 18.58 m.
The values in brackets indicate the amount of the current product normalized to the maximum value.
Select maxima
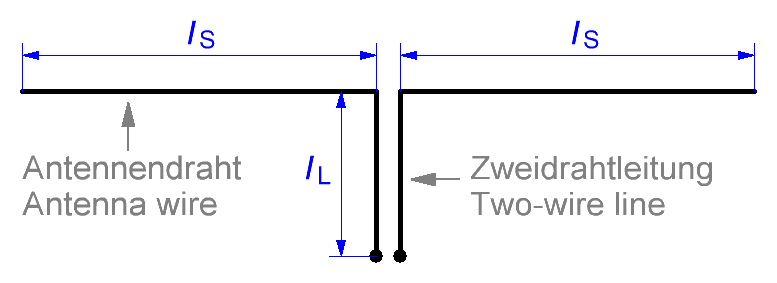 From the maxima, the electrical lengths of the antenna wire and the feedline can be calculated.
Since several maximas are always output, a maximum can be used to calculate the antenna wire length and the difference up to the next maximum for the calculation of the feedline.
The maxima do not have to be the first and the second.
It is also the second maximum for determining the length of the antenna wire and the difference up to the fourth maximum for the length of the feedline usable.
The choice depends solely on the installation possibilities (mast height, clamping points) of the antenna.
I chose from the calculated maxima 5.51 m and 12.58 m.
From the maxima, the electrical lengths of the antenna wire and the feedline can be calculated.
Since several maximas are always output, a maximum can be used to calculate the antenna wire length and the difference up to the next maximum for the calculation of the feedline.
The maxima do not have to be the first and the second.
It is also the second maximum for determining the length of the antenna wire and the difference up to the fourth maximum for the length of the feedline usable.
The choice depends solely on the installation possibilities (mast height, clamping points) of the antenna.
I chose from the calculated maxima 5.51 m and 12.58 m.
The maximium M1 first reached from the end point of the wire is the electrically length lSE of the antenna wire, here 5.51 m.
The difference from here 7.07 m to the next selected maximum M2 is the electrical length lLE of the two-wire feedline.
Now calculate the mechanically lengths of the antenna wire lSM and the length of the two-wire feedline lLM from the electrically lengths taking into account the velocity factors.
The antenna wires I use have a velocity factor of VFS = 0.98, the two-wire feedline has a velocity factor of VFL = 0.80 according to the manufacturer.
Thus, in the example, the lengths of the two halves of the doublet antenna resulted in 5.51 m (together 11.02 m) and the length of the two-wire feedline 5.66 m.
Using 2 maxima has the desired positive side effect.
Since the two antenna wire halves each have low impedances, the radiator halves can be connected directly to the antenna tuner even without a feedline.
If a two-wire feedline is required, the selected length together with the antenna wire also has a low impedance.
Control
All that remains is whether the lengths determined in this way also result in the expected low impedances on the selected bands.
The electrically length of the antenna wire halves (S) and the electrical length of the construction connected by the antenna and the feedline (S+Z) shall not be an integer multiples of ½ λ.
In the final step, therefore, the determined lengths are checked and the corresponding wavelengths are output.
The frequencies as well as the lengths of the antenna wire halves and two-wire feedline are from the two previous calculations.
Opportunities and limits
With the calculations shown here, only usable antenna wire lengths and feedline lengths can be determined.
Inferences about the radiation pattern and thus the effectiveness of the antennas are not possible.
In addition, the foot-point impedance will always vary somewhat according to the height of the construction and the existing buildings around.
But these changes should be able to compensate the already necessary antenna tuner.
Literature:
[1] Karl H. Hille, DL1VU: Windom- und Stromsummen-Antennen. Theuberger Verlag, Berlin 2000; Box 73, Order-no. X-9141